Forces
The commands in the Forces menu and Forces toolbar add, measure, and configure kinetostatic forces*. We will use the generic term Forces.
In MechDesigner, to calculate correctly the Forces that act on a Part:
•the kinematic-chain that is kinematically-defined,
•a minimum of one Part in the kinematic-chain has Mass, and usually a Mass Moment of Inertia - see Mass Properties, below
•the Power flows correctly through the kinematic-chain from the correct Power Source - see Configure Power Source.
* Norton calls it the “Inverse Dynamics Problem”.
Mass Properties
Parts have three sources of Mass and a Mass Moment of Inertia. The sources are: •Profile / Extrusions - see Extrusion > Mass Properties •User Mass Properties - see CAD-Line > Mass Properties tab > User Mass Properties •SolidWorks Mass Properties - see CAD-Line > Mass Properties tab > SOLIDWORKS Mass Properties We add together the three sources of Mass Properties. Note: If a Part has Mass but does not have Mass Moment of Inertia, it is called a Point-Mass (or a Black Hole!). In reality, a Mass is distributed, and so the body must also have a Mass Moment of Inertia. However, you can, if you wish, add a Point-Mass to a Part without a Mass Moment of Inertia. For very slow moving machines, or for Parts that mainly translate (rotate only a small amount), this may provide the accuracy you need. |
![]() On-line Tutorial: Tutorial 13: Forces Introduction
On-line Tutorial: Tutorial 13: Forces Introduction
Force menu

Forces menu (MD17)
Force toolbar
The Force toolbar is to the right of the graphics-area.
Buttons to Scale Force and Torque Vectors in the graphics-area.
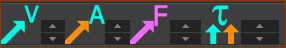
Vector scaling
F: Force Vectors, T: Torque Vectors
“Force Vectors” include both the Force Vectors and Torque Vectors.
If, after you display Force Vectors, the arrowheads of the vectors are outside of the graphics-area, you must use the Scale buttons to decrease the length of the Force Vectors and/or Torque Vectors - see Feedback Area > Vector Scaling buttons
Force definitions - as defined by IFTOMM International Federation on the Theory of Machines and Mechansims :
FORCE |
|---|
Action of its surroundings on a body tending to change its state of rest or motion. |
LINE OF ACTION OF A FORCE |
The line along which the vector that represents a given force lies. |
MAGNITUDE OF A FORCE |
Number of units of force obtained by comparing a given force with a standard, taken as unit force -(SI units : Newtons) |
ACTIVE [APPLIED] FORCE |
Force capable of producing motion. |
REACTION |
Force arising in a constraint and acting upon a constrained body due to the action of an active force upon that body. |
CENTRIPETAL FORCE |
Force causing the centripetal acceleration of a particle. |
INERTIA FORCE |
Product of the mass of a particle and the negative of its acceleration. Following D'Alembert, the inertia force can be regarded as being in equilibrium with the resultant of all the forces acting on the particle. |
CENTRIFUGAL FORCE |
Inertia force of a particle moving uniformly along a circular path. |
CORIOLIS FORCE |
Inertia force equal to the product of the mass of a particle and the negative of its Coriolis component of acceleration. |
GRAVITATIONAL FORCE |
Force equal to the product of the mass of a particle and the Gravitational Acceleration on Earth - taken as 9.806m/s/s. |
We calculate for you those Forces that result from these Accelerations :
CORIOLIS ACCELERATION |
|---|
Component of the absolute acceleration of a point due to its velocity relative to a rotating frame of reference. It equals twice the vector product of the angular velocity of the moving frame of reference and the relative velocity of the given moving point. |
CENTRIPETAL ACCELERATION |
Acceleration of a point towards the center of curvature of its path as it moves along a fixed curve. |
TANGENTIAL ACCELERATION |
Component of acceleration of a point collinear with its velocity. |
NORMAL ACCELERATION |
Component of acceleration of a point normal to its velocity. |
ANGULAR ACCELERATION |
Rate of change of angular velocity with respect to time. |